06-05 Tomographic (2D) Slices

In an imaging experiment, definition and selection of a virtual slice through the examined object or a patient are of great importance. They are determined by characteristics of the excitation pulse. One distinguishes between shaped and hard pulses (Figure 01-08).

06-05-01 Slice Definition

In analytical NMR studies, the maximal RF power is applied for a time suf­fi­ci­ent­ly long to give the desired pulse angle (hard pulse). In more complicated ex­pe­ri­ments, it is necessary to adjust the pulse amplitude with time so as to give a bet­ter defined frequency content (shaped pulse).

The pulse shape is used to give an approximately rectangular slice profile for the slices in the imaging experiments (Gaussian and sinc pulses; see Figure 01- 09) and can heavily influence image contrast in magnetic resonance imaging.

The phase of the RF pulse is also determined at this stage, with many MR ma­chi­nes only allowing phases of 0°, 90°, 180° or 270° to be selected. The re­sul­ting excitation pulses can be as short as 10 ms for non-selective hard pulses, and ty­pi­cal­ly a few milliseconds for the frequency-selective shaped pulses used in mag­ne­tic resonance imaging with peak-to-peak amplitudes of up to several hun­dred volts.

06-05-02 Slice Selection

We can express the gradient strength in either mT/m or in Hz/m. Since the pulse has a fixed bandwidth (provided that the pulse duration is held constant), raising the gradient strength increases the number of Hz/m; this results in a decrease in slice thickness (Figure 06-15).

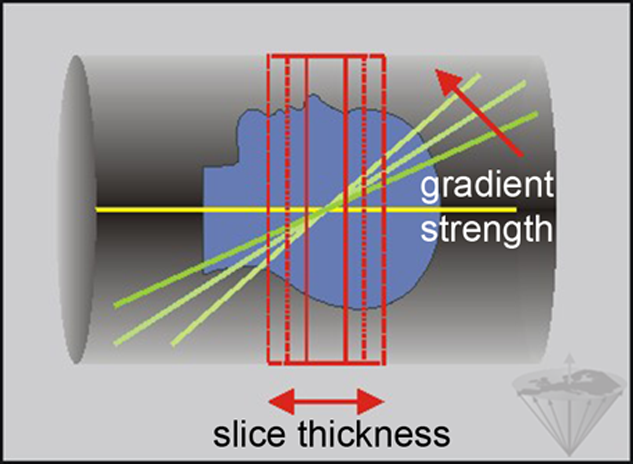
Figure 06-15: Slice thickness: moving the gradient in the direction of the arrow increases the number of Hz/m, and thus gradient strength. It decreases slice thickness.

For example, for a sinc pulse with a bandwidth of 2 kHz, increasing the slice gradient from 4 mT/m (1.7 kHz/cm) to 8 mT/m (3.4 kHz/cm) reduces the slice thickness from 11.8 mm to 5.9 mm.

Applying an RF pulse in the absence of any field gradients will excite the whole sample. If a field gradient is applied at the same time as the pulse, the magnetic field, and therefore the resonance frequency, will change with position within the sample. For an RF pulse at the resonance frequency excitation will oc­cur at the magnet center where the gradient has no effect (compare with Figure 06-05). Off-center, the nuclei cannot be excited by RF pulses at the Larmor fre­quen­cy.

The distance (or slice thickness) over which the nuclei in the center resonate is determined by the range of frequencies (bandwidth) contained in the excitation pulse and the strength of the field gradient. If the RF pulse contains only a well defined band of frequencies, then excitation will occur for a well defined range of positions. This excitation corresponds to the selection of a slice in the sample.

The length of the RF pulse, and thus also its bandwidth, is the se­cond factor influencing the slice thick­ness. The longer the pulse, the thin­ner the slice will be (Figure 06- 16). The trade-off for thinner slices is the prolongation of the echo time (TE). Because TE is measured from the center of the pulse, longer pul­ses for thinner slices mean a lon­ger initial TE, which, in turn, influences imaging time, image artifacts, and contrast. Figure 06-16: Slice thickness: in (a), long sinc pulses lead to thin slices whereas in (b), short sinc pulses increase slice thickness.

Changing the frequency of the RF pulse corresponds to moving the position of the nuclei on resonance from the center of the sample. In this way we can move the slice to any desired location along the axis (Figure 06-17). For a transverse slice, the slice gradient is applied along the z-axis; for a coronal slice, the slice gradient is applied along the y-axis; and for a sagittal slice, it is applied along the x-axis.

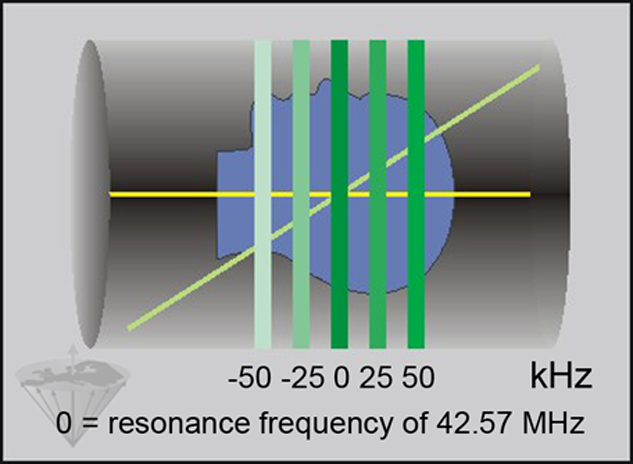
Figure 06-17: Moving the slice position: at 1.0 T, the resonance frequency in the center of the sample corresponds to 42.57 MHz. Changing the pulse frequency by several kHz moves the slice off-center.